Editor's note: This is the fourth article in a five-article series on central-chiller-plant modeling. The first three articles - "Primary/Secondary vs. Primary-Only Pumping" (http://bit.ly/Nelson_1), "Efficient Control of a Primary/Secondary Plant" (http://bit.ly/Nelson_2), and "Efficient Control of a Primary-Only Plant" (http://bit.ly/Nelson_3) - appeared in the April, May, and July 2011 issues of HPAC Engineering, respectively.
Low-load delta-T is said1 to exist in nearly every large distributed chilled-water system. The first three articles in this series2,3,4 described strategies for coping with low-load delta-T in plants with primary/secondary (P/S) and primary-only (P-only) distribution pumping. This article will discuss why low-load delta-T is so pervasive in central chiller plants and introduce a means of forcing load delta-T to 10°F.
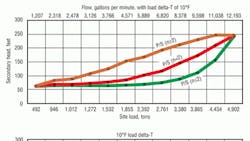
Non-uniform Secondary Flow
The top chart in Figure 1 illustrates a concept5 regarding the changing characteristics of secondary head as a function of loads active close to or far from a plant. The analysis is with P/S pumping and a load delta-T of 10°F. The general equation5 is:
H = Ch + Hd (Q ÷ Qd)n
where:
Ch = static head or constant pressure (60 ft assumed for model)
Hd = system head at design flow (245 ft - see Figure 4 of Article 1 in this series2)
Q = intermediate (secondary) flow in the system, gallons per minute
Qd = design flow of the system (12,193 gpm - see Figure 4 of Article 1 in this series2)
n = system-friction coefficient (2 for all analysis in the first three articles in this series2,3,4)
This equation was applied to distribution piping and an air-handler coil.
System-friction coefficient can vary from about 0.37 to 3.5,5 generating a system head area (Figure 1). System curves are given5 as system head vs. flow, as shown by the second horizontal axis in the top chart of Figure 1. With flow for each condition of n nearly identical, model data will be presented here as a function of site load, as shown by the primary horizontal axis in the top chart of Figure 1, to be consistent with most other charts in this series of articles.2,3,4
The bottom chart in Figure 1 is the same curve, with the axis reversed to agree with all other charts in this series of articles.2,3,4 Figure 1 shows system head can more than double as a function of the changing flow characteristics of a load. Each system, however, has its own characteristics of system head area,5 which may be more or less than presented in Figure 1.
Effect of Non-uniform Flow
Figure 2 illustrates the effect of non-uniform secondary flow on pump and plant performance. The top chart gives the secondary-pump power required to maintain a load delta-T of 10°F. At a site load of 3,380 tons, secondary-pump power varies from about 190 to 470 kw as the system head curve changes. If secondary-pump power were controlled with a differential-pressure transmitter (DPT), operators likely would set the DPT to more or less match the secondary-pump-power requirements of the n≥2 curve; when the system operated within the system head curve at a lower value of n, low-load delta-T would occur.
The bottom chart in Figure 2 illustrates the variation in plant power per ton of site load for the system head curves of Figure 1. At a site load of 3,380 tons, plant performance varies from about 0.575 to 0.66 kw per ton. This shows DPT control of a secondary pump to be questionable and the definition of plant performance before a plant is built and operating impractical.
Potential Secondary-Pump Power
Figure 3 illustrates the potential for inefficient plant performance attributed to the assumed 700-kw installed capacity of the secondary pump (see Figure 4 of the first article2 in this series). The top chart illustrates the magnitude of the additional pump power available to drive load delta-T to below 10°F. If the secondary pump were operating at full capacity, load delta-T would be as shown by the second horizontal axis in the top chart, varying from 10°F at design conditions to 2.1°F at 492 site tons. At 3,380 site tons, about 310 kw of secondary-pump power would be required at n=2 conditions for a delta-T of 10°F to be provided; however, the DPT or operators potentially could call for 700 kw, driving load delta-T to 7.3°F. A lower delta-T would increase bypass flow and, thus, decrease plant efficiency, as shown by the bottom chart in Figure 3.
The bottom chart in Figure 3 illustrates plant power per ton of site load for the conditions in the top chart. Clearly, secondary-pump power can degrade plant performance significantly; therefore, DPT control of a pump is a potential source of low-load delta-T and inefficient plant performance. The bottom chart in Figure 2 illustrates plant performance for the three conditions of n for a delta-T of 10°F.
The bottom chart in Figure 3 also shows the performance of the P/S plant of the second article3 in this series (see Figure 6 of Article 23) with low-load delta-T and full loading of a chiller before another chiller is turned on (air-handler entering-water-temperature [(ewt)AH] control).
Secondary-Pump Power and Flow
According to the top chart in Figure 4, the pump power of the Article 23 plant falls between the 700-kw maximum and the power required to achieve 10°F delta-T for n=2 flow distribution. Load delta-T is shown by the secondary horizontal axis.
Page 2 of 2
The bottom chart in Figure 4 gives the flow for the full 700-kw pump power. Load delta-T is shown by the secondary horizontal axis. The P/S system with air-handler-entering-water-temperature control falls between the full-power flow and the flow required for a delta-T of 10°F.
High-Force Coil Valve
The top chart in Figure 5 shows no change in secondary-pump power with a high-force coil valve installed in the Article 23 plant¡¯s system. A high-force coil valve reduces secondary flow and provides a load delta-T of 10°F, as shown by the bottom chart.
The top chart in Figure 6 shows air-handler leaving-water temperature [(lwt)AH] for the Article 23 plant both with and without a high-force coil valve installed to overcome high secondary-pump power and provide air-handler leaving-water temperature of approximately 54°F. The bottom chart in Figure 6 shows the secondary head generated by the high-force coil valve. The top chart in Figure 5 shows secondary-pump power to be the same; therefore, any reduction in plant power can be attributed primarily to improved chiller performance.
P/S Plant With High-Force Coil Valve
Figure 7 shows the effect of the high-force coil valve on chiller and plant performance. Secondary-pump power is the same for the plant with the high-force coil valve and the one with the low-force coil valve. The top chart shows chiller performance is better with the high-force coil valve primarily because evaporator leaving-water temperature [(lwt)evap] is greater, as the high-force coil valve minimizes the mixing of return and supply water. The horizontal axis of the top chart shows evaporator leaving-water temperature for both coil valves, showing the chiller must provide water of less than 44°F with the low-force coil valve because of mixing with bypass water. The bottom chart illustrates the improvement in plant performance with the high-force coil valve, which forces the return water to 54°F and, thus, delta-T to 10°F. The improvement is attributed primarily to bypass-flow reduction.
P-Only Plant With High-Force Coil Valve
Figure 8 illustrates the effect of the high-force coil valve on the Article 34 P-only plant with evaporator-velocity control. The top chart illustrates the decrease in flow attributed to the high-force coil valve, which provides a load delta-T of 10°F. Also, the top chart shows evaporator leaving-water temperature to be about 44°F for both valves. The bottom chart gives evaporator velocity with the two valves, illustrating how the high-force coil valve reduces evaporator velocity because of the decreased flow, as shown in the top chart, and the number of chiller on, as shown by the horizontal axis. For three site loads, the high-force coil valve requires fewer chillers in operation, which results in lower velocity through the evaporator and, thus, less pump power, but also lower chiller performance.
Plant and Chiller Performance
The top chart in Figure 9 illustrates chiller performance, showing that the plant with the low-force valve performs a little better at site loads of 1,855, 1,012, and 946 tons. This is attributed to the number of chillers on, as shown by the horizontal axis in the top chart in Figure 9 and by the bottom chart in Figure 8. More chillers are on in the low-force-coil-valve plant to eliminate high evaporator velocities, as discussed in Article 3.4 The high-force coil valve drives load delta-T to 10°F and, therefore, reduces velocity through the evaporator. The bottom chart illustrates the improved plant performance with the high-force coil valve, with improvement occurring when fewer chillers are on.
Plant-Performance Summary
Figure 10 shows the performance of the four plants considered in this article. The high-force coil valve improved the performance of both the P/S plant and the P-only plant. For the site loads considered, the P/S plant performs a little better than the P-only plant. The performance of the considered plants, however, generally is about the same, with no clear advantage for any of the four. Keep in mind that all of the plants in Figure 10 are overpumped, and the result of overpumping is decreased plant performance, especially at reduced site loads. The most effective way to improve plant performance is to eliminate low-load delta-T by decreasing excessive distribution pumping.
Summary
Through this analysis, we learned:
- Non-uniform secondary flow could explain some of the difficulty in controlling load delta-T in central systems.
- Maintaining minimum required secondary-pump power is a must for efficient plant operation.
- The performance of the P/S plant of Article 23 improves - albeit slightly - with the high-force coil valve because delta-T is forced to 10°F, which reduces flow in the bypass.
- For the Article 34 P-only plant with evaporator-velocity control, primary flow decreases with the high-force coil valve. Fewer chillers are required to be on, which results in lower evaporator velocity.
- With the high-force coil valve, the performance of the Article 34 P-only plant is as great as, if not greater than, it is with the low-force coil valve primarily because of the number of chillers on and the chiller-load percentage. Chiller performance, however, is degraded, unlike in the P/S plant.
Conclusion
Characteristics of load can cause low-load delta-T. Setting a DPT to provide 10°F delta-T is a challenge, if not impossible. Overpumping a secondary pump causes low-load delta-T. Installing a high-force coil valve has a positive effect for given secondary-pump power. If excessive secondary-pump power exists, however, the result generally is inefficient plant operation. Figure 10 shows the P/S plant with high-force coil valve performs best for the loads and conditions considered, with the P-only plant close behind. The next and final article in this series will discuss secondary-pump control with something other than a DPT.
References
1) Kirsner, W. (1996, November). The demise of the primary-secondary pumping paradigm for chilled water plant design. Heating/Piping/Air-Conditioning, pp. 73-75, 77, 78.
2) Nelson, K. (2011, April). Primary/secondary vs. primary-only pumping. HPAC Engineering, pp. 34-40. Available at http://bit.ly/Nelson_1
3) Nelson, K. (2011, May). Efficient control of a primary/secondary plant. HPAC Engineering, pp. 34, 37-41. Available at http://bit.ly/Nelson_2
4) Nelson, K. (2011, July). Efficient control of a primary-only plant. HPAC Engineering, pp. 32, 34, 36-39. Available at http://bit.ly/Nelson_3
5) Rishel, J.B. (2001, February). Applying affinity laws for centrifugal pumps. HPAC Engineering, pp. 35-38.
Kirby Nelson, PE, has been involved in the modeling of HVAC systems since the oil embargo of 1973 - first as corporate energy manager for Texas Instruments Inc., then as a consultant. Models he has used include DOE-2, E Cube, and models developed on an analog/digital computer, including models of cleanrooms. A life member of the American Society of Heating, Refrigerating and Air-Conditioning Engineers, he has presented numerous papers, led an energy engineering delegation to China, and more recently developed models for district cooling systems, thermal-storage systems, and central plants.
Did you find this article useful? Send comments and suggestions to Executive Editor Scott Arnold at [email protected].